pm_sampleCCF::setACF Interface Reference

Return the auto-correlation function (ACF) \((f\star f)(\tau)\) of the discrete signal \(f\) lagging itself for a range of lags that spans the sequence length.
More...

Detailed Description

Return the auto-correlation function (ACF) \((f\star f)(\tau)\) of the discrete signal \(f\) lagging itself for a range of lags that spans the sequence length.

See the documentation of the parent module pm_sampleCCF for algorithmic details and sample correlation matrix definition.
Note that acf is always real-valued at lag 0 by definition, even when the input f is of type complex.
For all non-zero lags, the imaginary component of the auto-correlation function is an odd function.

Warning

This low-level generic interface neither right-pads nor normalizes the input sequence in any form.
The input sequence is used as is for computing the ACF.
In general, the following steps should be taken before and after computing the ACF using this routine,

1. If the original (unpadded) sequence is not already mean-shifted (with zero mean), it is highly recommended to mean-shift the sequence first before computing the ACF.
   This can be readily done by the routines of pm_sampleMean and pm_sampleShift.
   
2. While the input mean-shifted sequence can be passed to this generic interface as is, it is highly recommended to right-pad the mean-shifted sequence by zeros to the minimum length of size(f) * 2 - 1.
   This means that the length of the input sequence should always be an odd number, although not necessarily, if you know what you are doing.
   
3. The output acf must from the generic interface must be multiplied by 1 / f(1) to properly normalize the output acf to the range [-1, +1].
   While easy, this can also be readily done via the generic interfaces of pm_sampleScale.
   

Note

When the input sequence is right-padded such that size(acf) = size(f) * 2 - 1, then,

1. the resulting slice acf(1 : size(f)) by this generic interface contains the auto-correlation corresponding to lags [(i, i = 0, size(f))].
2. the resulting slice acf(size(f) + 1 : size(acf)) contains the auto-correlation corresponding to lags [(i, i = -size(f) + 1, -1)].

Parameters

[in]	factor	: The input contiguous vector of shape (:) of type integer of default kind IK, containing the factorization of the length of the input sequence f whose FFT is to be computed. This input argument along with the input argument coef is the direct output of getFactorFFT.
[in]	coef	: The input contiguous vector of shape (1:size(data)) of the same type and kind as the input argument f, containing the trigonometric look up table required for FFT of the specified sequence. This input argument along with factor is the direct output of getFactorFFT.
[in,out]	f	: The input contiguous vector of arbitrary size (of minimum 2) of, type complex of kind any supported by the processor (e.g., CK, CK32, CK64, or CK128), type real of kind any supported by the processor (e.g., RK, RK32, RK64, or RK128), containing the first sequence in the auto-correlation computation. On output, the contents of f are destroyed. If the output inf = .true., then f contains the resulting unnormalized ACF of the input sequence.
[out]	work	: The output contiguous vector of the same type, kind, and size as the input f, used as a workspace. If the condition inf = .false. holds on output, then work contains the resulting unnormalized ACF of the input sequence f.
[out]	inf	: The output scalar of type logical of default kind LK. If .true., the resulting ACF is stored in the output argument f upon return from the procedure. If .false., the resulting ACF is stored in the output argument work upon return from the procedure.

Possible calling interfaces ⛓

use pm_sampleCCF, only: setACF
 
call setACF(factor(:), coef(1:nseq), f(1:nseq), work(1:nseq), inf)

Warning

The condition 2 < size(f) must hold for the corresponding input arguments.
The condition size(f) == size(coef) must hold for the corresponding input arguments.
The condition size(f) == size(work) must hold for the corresponding input arguments.
These conditions are verified only if the library is built with the preprocessor macro CHECK_ENABLED=1.

The pure procedure(s) documented herein become impure when the ParaMonte library is compiled with preprocessor macro CHECK_ENABLED=1.
By default, these procedures are pure in release build and impure in debug and testing builds.

See also

getACF
setACF
getCor
setCor
getRho
setRho
getCov
setCov
setECDF
getMean
setMean
getShifted
setShifted
getVar
setVar

Example usage ⛓

program example
 
    use pm_kind, only: SK, IK, LK, RK
    use pm_io, only: getErrTableWrite
    use pm_io, only: display_type
    use pm_io, only: getFormat
    use pm_fftpack, only: allocatable
    use pm_fftpack, only: getFactorFFT
    use pm_sampleCCF, only: setACF
    use pm_sampleCCF, only: setCCF
    use pm_sampleCCF, only: stdscale
    use pm_arrayRange, only: getRange
    use pm_distUnif, only: getUnifRand
    use pm_distNorm, only: getNormLogPDF
    use pm_arrayPad, only: getPaddedr
    use pm_arrayFill, only: getFilled
    use pm_sampleShift, only: getShifted
    use pm_arrayResize, only: setResized
    use pm_arraySpace, only: getLinSpace
    use pm_sampleNorm, only: getNormed
    use pm_sampleMean, only: getMean
    use pm_sampleVar, only: getVar
 
    implicit none
 
    logical(LK) :: inf
    type(display_type) :: disp
    character(:), allocatable :: format
    integer(IK) :: nsam, itry, ntry = 1
    disp = display_type(file = "main.out.F90")
 
    call disp%skip()
    call disp%show("!%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%")
    call disp%show("!Compute the cross-correlation of two samples.")
    call disp%show("!%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%")
    call disp%skip()
 
    block
        use pm_kind, only: TKG => RKS ! All other real types are also supported.
        integer(IK), allocatable :: factor(:), lag(:)
        real(TKG), allocatable :: seq(:), f(:), g(:), acf(:), coef(:), range(:)
        real(TKG), parameter :: ZERO = 0._TKG
        call disp%skip()
        call disp%show("nsam = 41")
                        nsam = 41
        call disp%show("range = getLinSpace(-10., 10., nsam)")
                        range = getLinSpace(-10., 10., nsam)
        call disp%show("seq = sin(range)")
                        seq = sin(range)
        call disp%show("seq = getPaddedr(getShifted(seq, -getMean(seq)), nsam - 1, ZERO)")
                        seq = getPaddedr(getShifted(seq, -getMean(seq)), nsam - 1, ZERO)
        call disp%show("seq")
        call disp%show( seq )
        call disp%show("call setResized(acf, size(seq, 1, IK))")
                        call setResized(acf, size(seq, 1, IK))
        call disp%show("factor = getFactorFFT(seq, coef, allocatable)")
                        factor = getFactorFFT(seq, coef, allocatable)
        call disp%show("f = seq")
                        f = seq
        call disp%show("call setACF(factor, coef, f, acf, inf)")
                        call setACF(factor, coef, f, acf, inf)
        call disp%show("acf = merge(f, acf, inf) / size(acf)")
                        acf = merge(f, acf, inf) / size(acf)
        call disp%show("size(acf)")
        call disp%show( size(acf) )
        call disp%show("acf")
        call disp%show( acf )
        call disp%show("f = seq; g = seq")
                        f = seq; g = seq
        call disp%show("call setCCF(factor, coef, f, g, acf, inf) ! for comparison with the above.")
                        call setCCF(factor, coef, f, g, acf, inf) ! for comparison with the above.
        call disp%show("acf = merge(f, g, inf) / size(acf)")
                        acf = merge(f, g, inf) / size(acf)
        call disp%show("size(acf)")
        call disp%show( size(acf) )
        call disp%show("acf")
        call disp%show( acf )
        call disp%show("lag = getRange(-nsam + 1_IK, nsam - 1_IK)")
                        lag = getRange(-nsam + 1_IK, nsam - 1_IK)
        call disp%show("if (0 /= getErrTableWrite(SK_'setACF.crd.sin.RK.txt', reshape([range, seq], [nsam, 2_IK]), header = SK_'crd,f')) error stop 'acf outputting failed.'")
                        if (0 /= getErrTableWrite(SK_'setACF.crd.sin.RK.txt', reshape([range, seq], [nsam, 2_IK]), header = SK_'crd,f')) error stop 'acf outputting failed.'
        call disp%show("if (0 /= getErrTableWrite(SK_'setACF.acf.sin.RK.txt', reshape([real(lag, TKG), acf], [size(lag), 2]), header = SK_'lag,acf')) error stop 'acf outputting failed.'")
                        if (0 /= getErrTableWrite(SK_'setACF.acf.sin.RK.txt', reshape([real(lag, TKG), acf], [size(lag), 2]), header = SK_'lag,acf')) error stop 'acf outputting failed.'
        call disp%skip()
    end block
 
end program example

Example Unix compile command via Intel ifort compiler ⛓

#!/usr/bin/env sh
rm main.exe
ifort -fpp -standard-semantics -O3 -Wl,-rpath,../../../lib -I../../../inc main.F90 ../../../lib/libparamonte* -o main.exe
./main.exe

Example Windows Batch compile command via Intel ifort compiler ⛓

del main.exe
set PATH=..\..\..\lib;%PATH%
ifort /fpp /standard-semantics /O3 /I:..\..\..\include main.F90 ..\..\..\lib\libparamonte*.lib /exe:main.exe
main.exe

Example Unix / MinGW compile command via GNU gfortran compiler ⛓

#!/usr/bin/env sh
rm main.exe
gfortran -cpp -ffree-line-length-none -O3 -Wl,-rpath,../../../lib -I../../../inc main.F90 ../../../lib/libparamonte* -o main.exe
./main.exe

Example output ⛓

 
!%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%
!Compute the cross-correlation of two samples.
!%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%
 
 
nsam = 41
range = getLinSpace(-10., 10., nsam)
seq = sin(range)
seq = getPaddedr(getShifted(seq, -getMean(seq)), nsam - 1, ZERO)
seq
+0.544021070, +0.751511455E-1, -0.412118465, -0.798487127, -0.989358246, -0.937999964, -0.656986594, -0.215119958, +0.279415518, +0.705540299, +0.958924294, +0.977530122, +0.756802440, +0.350783259, -0.141119972, -0.598472178, -0.909297407, -0.997494996, -0.841470957, -0.479425520, +0.261678927E-7, +0.479425579, +0.841470957, +0.997494996, +0.909297407, +0.598472178, +0.141120031, -0.350783199, -0.756802440, -0.977530122, -0.958924294, -0.705540299, -0.279415458, +0.215120018, +0.656986594, +0.937999964, +0.989358246, +0.798487127, +0.412118524, -0.751510859E-1, -0.544021130, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000, +0.00000000
call setResized(acf, size(seq, 1, IK))
factor = getFactorFFT(seq, coef, allocatable)
f = seq
call setACF(factor, coef, f, acf, inf)
acf = merge(f, acf, inf) / size(acf)
size(acf)
+81
acf
+19.4603882, +16.5995274, +9.90436745, +1.18769729, -7.34153843, -13.6373682, -16.3073692, -14.9171066, -10.0427866, -3.07247305, +4.18143559, +9.95184994, +12.9474525, +12.6391058, +9.33939934, +4.06805849, -1.74958324, -6.66454172, -9.56497478, -9.92598820, -7.89280128, -4.18799973, +0.120422743, +3.91993761, +6.34000349, +6.95056915, +5.82759619, +3.47926354, +0.664440393, -1.83813798, -3.44246578, -3.89219093, -3.28998709, -2.02030611, -0.597234249, +0.511142671, +1.02663577, +0.930730164, +0.442753345, -0.817697793E-1, -0.295960993, -0.295958340, -0.817682743E-1, +0.442753911, +0.930729270, +1.02663636, +0.511143804, -0.597234249, -2.02030659, -3.28998709, -3.89218998, -3.44246483, -1.83813930, +0.664440751, +3.47926354, +5.82759619, +6.95056915, +6.34000349, +3.91993928, +0.120418593, -4.18800020, -7.89280176, -9.92598629, -9.56497478, -6.66454268, -1.74958158, +4.06805849, +9.33940029, +12.6391058, +12.9474497, +9.95185184, +4.18143511, -3.07247376, -10.0427876, -14.9171066, -16.3073692, -13.6373672, -7.34153557, +1.18769836, +9.90436840, +16.5995255
f = seq; g = seq
call setCCF(factor, coef, f, g, acf, inf) ! for comparison with the above.
acf = merge(f, g, inf) / size(acf)
size(acf)
+81
acf
+19.4603882, +16.5995274, +9.90436745, +1.18769729, -7.34153843, -13.6373682, -16.3073692, -14.9171066, -10.0427866, -3.07247305, +4.18143559, +9.95184994, +12.9474525, +12.6391058, +9.33939934, +4.06805849, -1.74958324, -6.66454172, -9.56497478, -9.92598820, -7.89280128, -4.18799973, +0.120422743, +3.91993761, +6.34000349, +6.95056915, +5.82759619, +3.47926354, +0.664440393, -1.83813798, -3.44246578, -3.89219093, -3.28998709, -2.02030611, -0.597234249, +0.511142671, +1.02663577, +0.930730164, +0.442753345, -0.817697793E-1, -0.295960993, -0.295958340, -0.817682743E-1, +0.442753911, +0.930729270, +1.02663636, +0.511143804, -0.597234249, -2.02030659, -3.28998709, -3.89218998, -3.44246483, -1.83813930, +0.664440751, +3.47926354, +5.82759619, +6.95056915, +6.34000349, +3.91993928, +0.120418593, -4.18800020, -7.89280176, -9.92598629, -9.56497478, -6.66454268, -1.74958158, +4.06805849, +9.33940029, +12.6391058, +12.9474497, +9.95185184, +4.18143511, -3.07247376, -10.0427876, -14.9171066, -16.3073692, -13.6373672, -7.34153557, +1.18769836, +9.90436840, +16.5995255
lag = getRange(-nsam + 1_IK, nsam - 1_IK)
if (0 /= getErrTableWrite(SK_'setACF.crd.sin.RK.txt', reshape([range, seq], [nsam, 2_IK]), header = SK_'crd,f')) error stop 'acf outputting failed.'
if (0 /= getErrTableWrite(SK_'setACF.acf.sin.RK.txt', reshape([real(lag, TKG), acf], [size(lag), 2]), header = SK_'lag,acf')) error stop 'acf outputting failed.'
 
 

Postprocessing of the example output ⛓

#!/usr/bin/env python
 
import matplotlib.pyplot as plt
import pandas as pd
import numpy as np
import glob
import sys
 
linewidth = 2
fontsize = 17
 
for kind in ["sin.RK"]:
 
    file = glob.glob("*crd*"+kind+".txt")[0]
    df = pd.read_csv(file, delimiter = ",")
 
    #print(df.values)
    fig = plt.figure(figsize = (8, 6))
    ax = plt.subplot(1,1,1)
    ax.plot ( df.values[:, 0]
            , df.values[:,1:]
            , zorder = 1000
            )
    plt.minorticks_on()
    ax.set_xlabel("x", fontsize = 17)
    ax.set_ylabel("f(x)", fontsize = 17)
    ax.tick_params(axis = "x", which = "minor")
    ax.tick_params(axis = "y", which = "minor")
    plt.grid(visible = True, which = "both", axis = "both", color = "0.85", linestyle = "-")
    ax.legend([file.split(".")[-3] + "(x)"], fontsize = fontsize)
    plt.tight_layout()
    plt.savefig(file.replace(".txt",".png"))
 
    file = glob.glob("*acf*"+kind+".txt")[0]
    df = pd.read_csv(file, delimiter = ",")
    fig = plt.figure(figsize = (8, 6))
    ax = plt.subplot(1,1,1)
    ax.plot ( df.values[:, 0]
            , df.values[:,1]
            , zorder = 1000
            )
    plt.minorticks_on()
    ax.set_xlabel("Lag", fontsize = 17)
    ax.set_ylabel("acf(f)", fontsize = 17)
    ax.tick_params(axis = "x", which = "minor")
    ax.tick_params(axis = "y", which = "minor")
    plt.grid(visible = True, which = "both", axis = "both", color = "0.85", linestyle = "-")
    plt.tight_layout()
    plt.savefig(file.replace(".txt",".png"))

Visualization of the example output ⛓

Test:

test_pm_sampleCCF

Internal naming convention:

The following illustrates the internal naming convention used for the procedures within this generic interface.

setACF_FP_D1_CK5()
   ||| || || |||
   ||| || || The type and kind parameters of the input sequence.
   ||| || The dimension of the input sequence `f`.
   ||| The method used: FP => fftpack.
   ACF: Cross-Correlation Function.

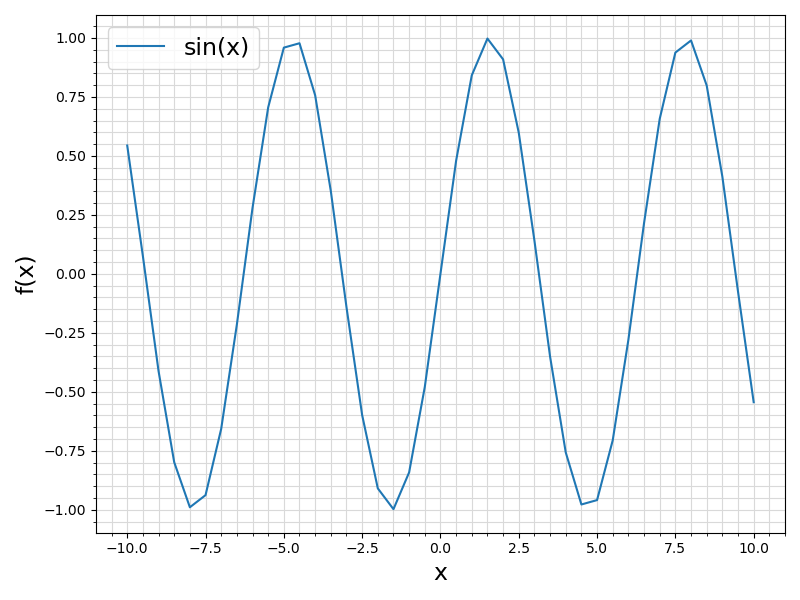
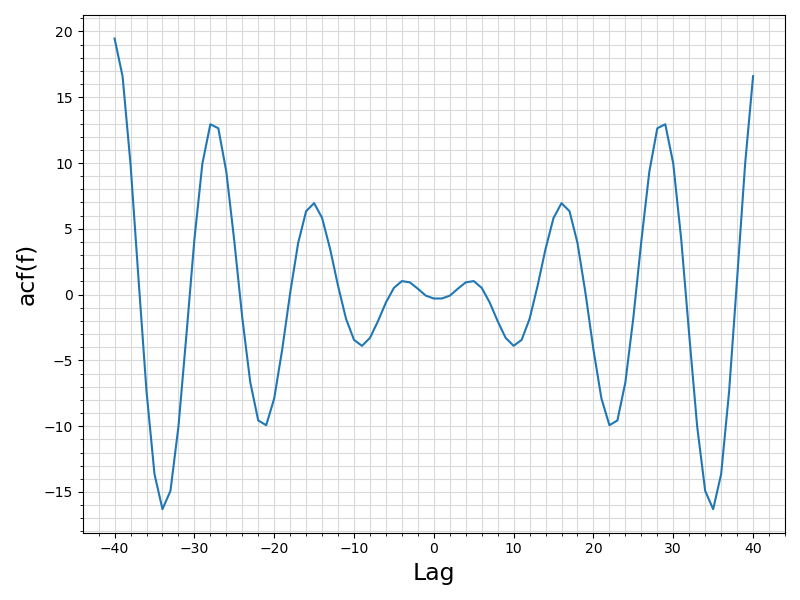
Final Remarks ⛓

---

If you believe this algorithm or its documentation can be improved, we appreciate your contribution and help to edit this page's documentation and source file on GitHub.
For details on the naming abbreviations, see this page.
For details on the naming conventions, see this page.
This software is distributed under the MIT license with additional terms outlined below.

1. If you use any parts or concepts from this library to any extent, please acknowledge the usage by citing the relevant publications of the ParaMonte library.
   
2. If you regenerate any parts/ideas from this library in a programming environment other than those currently supported by this ParaMonte library (i.e., other than C, C++, Fortran, MATLAB, Python, R), please also ask the end users to cite this original ParaMonte library.
   

This software is available to the public under a highly permissive license.
Help us justify its continued development and maintenance by acknowledging its benefit to society, distributing it, and contributing to it.

Copyright

Computational Data Science Lab

Author:

Fatemeh Bagheri, Monday 02:15 AM, September 27, 2021, Dallas, TX
Amir Shahmoradi, Wednesday 4:13 AM, August 13, 2016, Institute for Computational Engineering and Sciences (ICES), The University of Texas at Austin

Definition at line 525 of file pm_sampleCCF.F90.

---

The documentation for this interface was generated from the following file:

• src/fortran/main/pm_sampleCCF.F90