|
ParaMonte MATLAB 3.0.0
Parallel Monte Carlo and Machine Learning Library
See the latest version documentation. |
|
ParaMonte MATLAB 3.0.0
Parallel Monte Carlo and Machine Learning Library
See the latest version documentation. |
Go to the source code of this file.
Functions | |
| function | getFunc (in x, in y) |
| Return the value of the 2-dimensional Himmelblau function at the specified input value. More... | |
| function getFunc | ( | in | x, |
| in | y | ||
| ) |
Return the value of the 2-dimensional Himmelblau function at the specified input value.
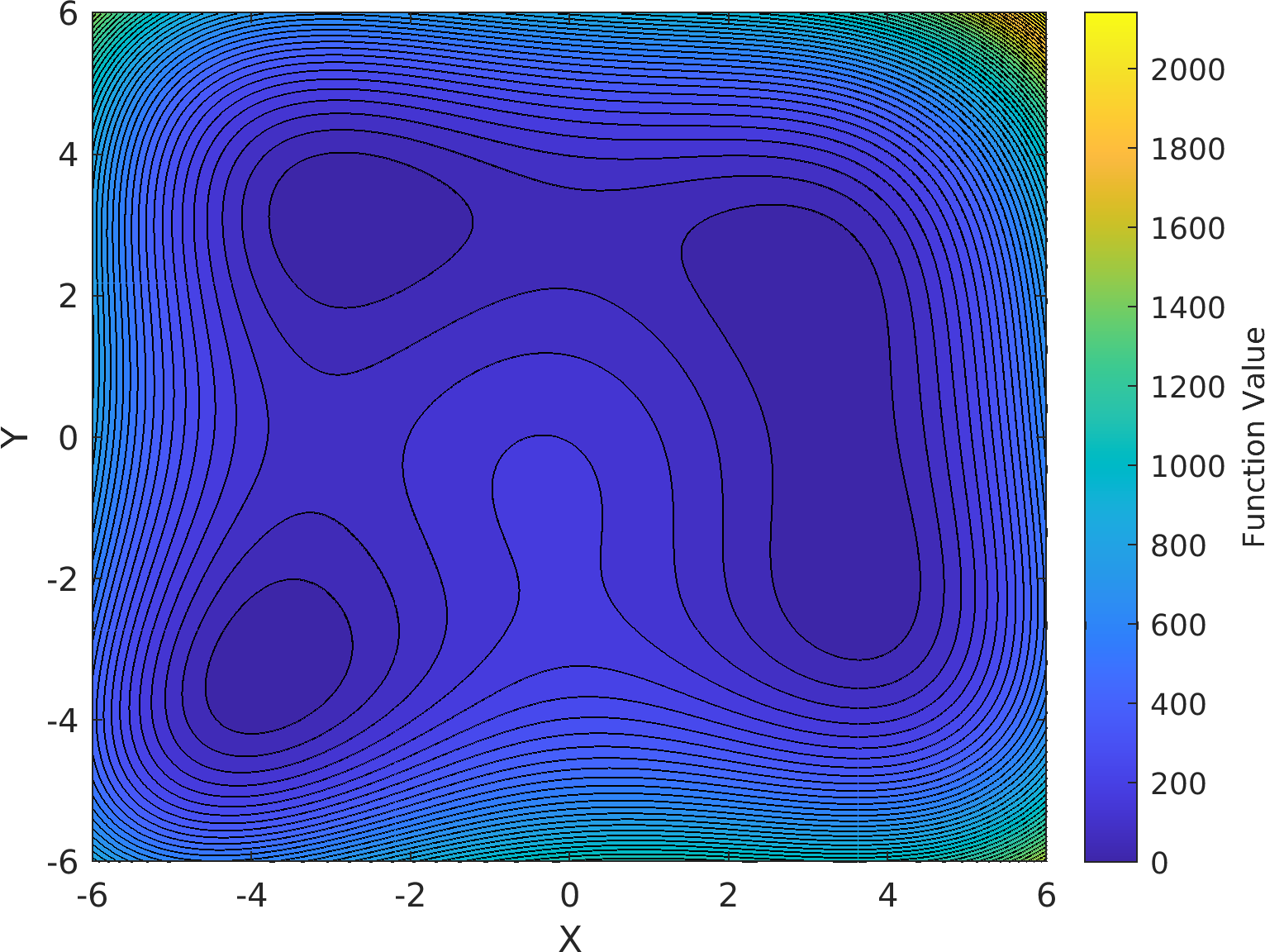
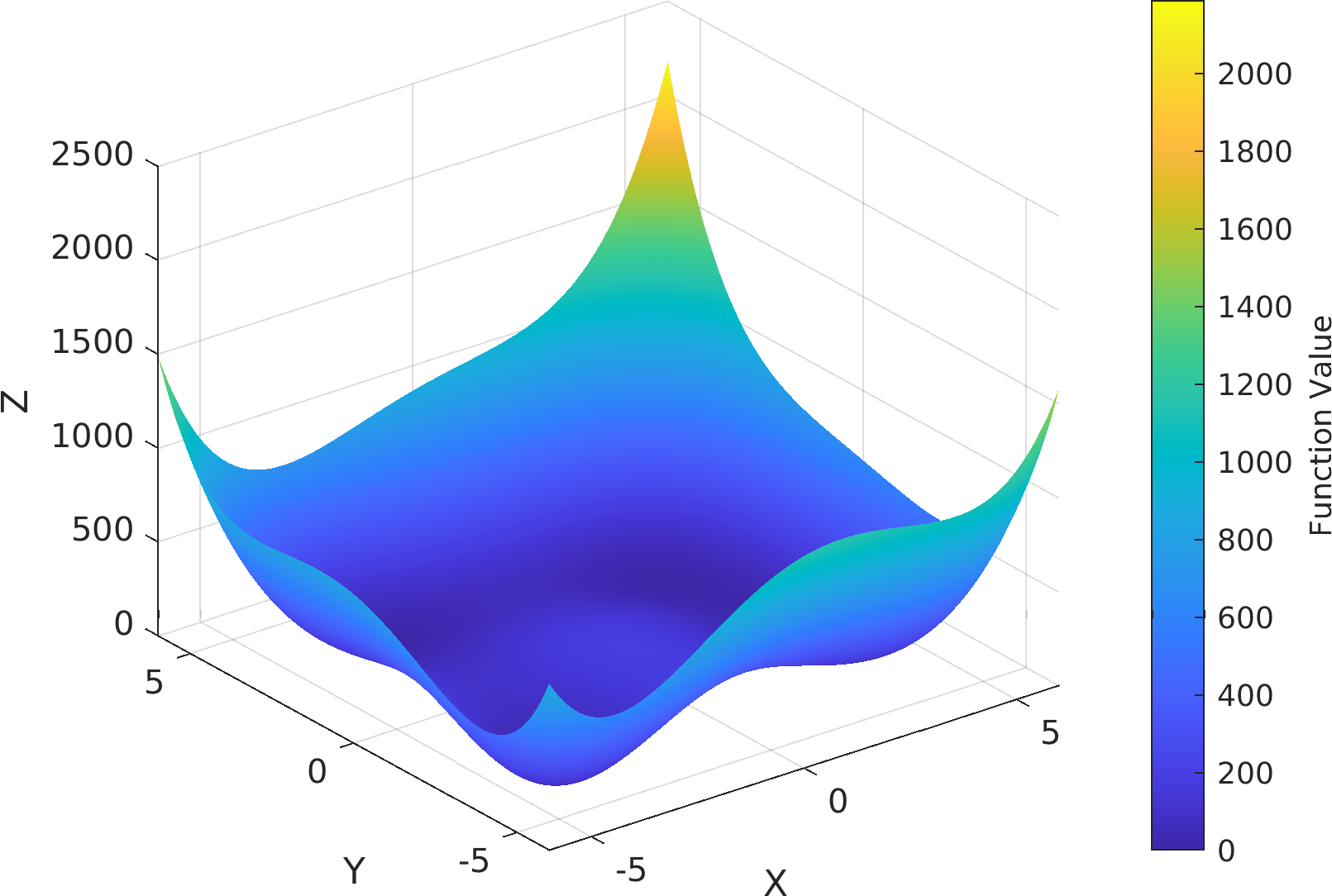
Himmelblau's function is a multi-modal function, used to test the performance of optimization algorithms. The function is defined by:
\begin{equation} H(x, y) = (x^{2} + y - 11)^{2} + (x + y^{2} - 7)^{2} ~. \end{equation}
It has one local maximum at x = -0.270845 and y = -0.923039 where H(x, y) = 181.617, and four identical local minima:
\begin{eqnarray} H(3.0,2.0) &=& 0.0 ~,\\ H(-2.805118, 3.131312) &=& 0.0 ~,\\ H(-3.779310, -3.283186) &=& 0.0 ~,\\ H(3.584428, -1.848126) &=& 0.0 ~. \end{eqnarray}
The function is named after David Mautner Himmelblau (1924–2011), who introduced it.
The locations of all the minima can be found analytically.
| [in] | x | : The input scalar or array of the same rank and shape as other input array-like arguments of type MATLAB double, representing the x-component of the state within the domain of Himmelblau function at which the function value must be computed. |
| [in] | y | : The input scalar or array of the same rank and shape as other input array-like arguments of type MATLAB double, representing the y-component of the state within the domain of Himmelblau function at which the function value must be computed. |
func : The output scalar or array of the same rank and shape as the input array-like arguments representing the value of the Himmelblau function at the specified input x and y.
Possible calling interfaces ⛓
Example usage ⛓
Final Remarks ⛓
If you believe this algorithm or its documentation can be improved, we appreciate your contribution and help to edit this page's documentation and source file on GitHub.
For details on the naming abbreviations, see this page.
For details on the naming conventions, see this page.
This software is distributed under the MIT license with additional terms outlined below.
This software is available to the public under a highly permissive license.
Help us justify its continued development and maintenance by acknowledging its benefit to society, distributing it, and contributing to it.