pm_distGeomCyclic::isFailedGeomCyclicFit Interface Reference

Generate and return .true. if the parameters of a least-squares fit to the histogram representing a Cyclic-Geometric-distributed sample can be successfully inferred, otherwise, return .false..
More...

Detailed Description

Generate and return .true. if the parameters of a least-squares fit to the histogram representing a Cyclic-Geometric-distributed sample can be successfully inferred, otherwise, return .false..

See the documentation of pm_distGeomCyclic for more details on the Cyclic Geometric distribution.
Given a set of Cyclic Bernoulli trial success steps \(\ms{stepSuccess}\) and the corresponding number of successes \(\ms{freqSuccess}\) at these steps, with the supplied Cyclic Bernoulli trial \(\ms{period}\), the procedures of this generic interface return the best-fit parameters \((\ms{probSuccess}, \ms{normFac})\) of the following curve,

\begin{eqnarray} \large \ms{freqSuccess} &=& \ms{normFac} \times \pi_{\mathcal{CG}} (X = \ms{stepSuccess} ~|~ \ms{probSuccess}, \ms{period}) ~, \nonumber \\ &=& \ms{normFac} \times \frac{\ms{probSuccess} (1 - \ms{probSuccess})^{\ms{stepSuccess} - 1}}{1 - (1 - \ms{probSuccess})^{\ms{period}}} ~, \end{eqnarray}

where the probability of success on each trial is \(\ms{probSuccess}\), and \(\pi_{\mathcal{CG}}\) is the probability mass function of the \(\ms{stepSuccess}\)th trial being the first success in a cyclic trial set with the cycle \(\ms{period}\).

Note

This fitting appears frequently in Fork-Join parallel MCMC simulations of the ParaMonte library.
See Amir Shahmoradi, Fatemeh Bagheri (2020). ParaDRAM: A Cross-Language Toolbox for Parallel High-Performance Delayed-Rejection Adaptive Metropolis Markov Chain Monte Carlo Simulations. for details and discussion.

Parameters

[in]	stepSuccess	: The input contiguous positive vector of type real of the same kind as that of probSuccess, type integer of default kind IK, containing the steps ( \(x\)) at which contribution frequencies represented by input vector freqSuccess have occurred.
[in]	freqSuccess	: The input contiguous positive vector of the same type, kind, and size as the input stepSuccess, containing the contribution frequencies at the corresponding stepSuccess in the histogram.
[in]	period	: The input positive scalar of type integer of default kind IK, containing the period of the Cyclic Geometric distribution. The period represents the maximum number of Bernoulli trials in the experiment. Note that the condition maxval(stepSuccess) <= period must hold and the sizes of stepSuccess and freqSuccess vectors must be smaller than the specified period.
[out]	probSuccess	: The output positive scalar of type real of kind any supported by the processor (e.g., RK, RK32, RK64, or RK128), representing the best-fit probability-of-success parameter of the Cyclic Geometric distribution inferred from the fitting.
[in]	normFac	: The output positive scalar of the same type and kind as the output probSuccess, containing the best-fit normalization constant of the histogram represented by the input stepSuccess and freqSuccess`.

Returns

failed : The output scalar of type logical of default kind LK that is .true. if and only if the best-fit output parameters are successfully inferred.
The algorithm can fail only if the optimizer fails to converge to set of best-fit parameters.

Possible calling interfaces ⛓

use pm_distGeomCyclic, only: isFailedGeomCyclicFit
 
failed = isFailedGeomCyclicFit(stepSuccess(1:period), freqSuccess(1:period), period, probSuccess, normFac)

Warning

The condition all(0 < stepSuccess) must hold for the corresponding input arguments.
The condition 1 < size(freqSuccess)) must hold for the corresponding input arguments.
The condition size(stepSuccess) == size(freqSuccess) must hold for the corresponding input arguments.
The condition all(stepSuccess <= size(stepSuccess)) must hold for the corresponding input arguments.
The condition all(stepSuccess <= period) must hold for the corresponding input arguments.
These conditions are verified only if the library is built with the preprocessor macro CHECK_ENABLED=1.

Remarks

The procedures under discussion are impure.

See also

setGeomCyclicRand
setGeomCyclicLogPMF
setGeomCyclicLogCDF

Example usage ⛓

program example
 
    use pm_kind, only: SK, IK
    use pm_arraySpace, only: getLinSpace
    use pm_distGeomCyclic, only: getGeomCyclicRand
    use pm_distGeomCyclic, only: isFailedGeomCyclicFit
    use pm_distGeomCyclic, only: getGeomCyclicLogPMF
    use pm_arrayUnique, only: setUnique
    use pm_io, only: getErrTableWrite
    use pm_io, only: display_type
 
    implicit none
 
    integer(IK) :: period, i
    type(display_type) :: disp
    integer(IK), allocatable :: rand(:)
    integer(IK), allocatable :: freqSuccess(:)
    integer(IK), allocatable :: stepSuccess(:)
    disp = display_type(file = "main.out.F90")
 
    block
        use pm_kind, only: RKG => RKH
        real(RKG) :: probSuccess, probSuccessFit, normFacFit
        real(RKG), allocatable :: x(:), y(:)
        call disp%skip()
        call disp%show("probSuccess = .1; period = 20")
                        probSuccess = .1; period = 20
        call disp%show("rand = getGeomCyclicRand(probSuccess, [(period, i = 1, 1000)])")
                        rand = getGeomCyclicRand(probSuccess, [(period, i = 1, 1000)])
        call disp%show("if (0 /= getErrTableWrite(file = 'isFailedGeomCyclicFit.rnd.txt', table = rand)) error stop 'table output failed.'")
                        if (0 /= getErrTableWrite(file = 'isFailedGeomCyclicFit.rnd.txt', table = rand)) error stop 'table output failed.'
        call disp%show("call setUnique(getGeomCyclicRand(probSuccess, [(period, i = 1, 1000)]), unique = stepSuccess, count = freqSuccess, order = -1_IK)")
                        call setUnique(getGeomCyclicRand(probSuccess, [(period, i = 1, 1000)]), unique = stepSuccess, count = freqSuccess, order = -1_IK)
        call disp%show("stepSuccess")
        call disp%show( stepSuccess , format = SK_"(sp,20(g0,:,', '))")
        call disp%show("freqSuccess")
        call disp%show( freqSuccess , format = SK_"(sp,20(g0,:,', '))")
        call disp%show("if (isFailedGeomCyclicFit(stepSuccess, freqSuccess, period, probSuccessFit, normFacFit)) error stop 'Fitting failed.'")
                        if (isFailedGeomCyclicFit(stepSuccess, freqSuccess, period, probSuccessFit, normFacFit)) error stop 'Fitting failed.'
        call disp%show("[probSuccessFit, normFacFit]")
        call disp%show( [probSuccessFit, normFacFit] )
        call disp%show("x = getLinSpace(1._RKG, real(period, RKG), 500_IK) ! for visualization.")
                        x = getLinSpace(1._RKG, real(period, RKG), 500_IK) ! for visualization.
        call disp%show("y = normFacFit * probSuccessFit * (1 - probSuccessFit)**(x - 1) / (1 - (1 - probSuccessFit)**period) ! for visualization.")
                        y = normFacFit * probSuccessFit * (1 - probSuccessFit)**(x - 1) / (1 - (1 - probSuccessFit)**period) ! for visualization.
        call disp%show("y = normFacFit * exp(getGeomCyclicLogPMF(x, probSuccessFit, period)) ! for visualization.")
                        y = normFacFit * exp(getGeomCyclicLogPMF(x, probSuccessFit, period)) ! for visualization.
        call disp%show("if (0 /= getErrTableWrite(file = 'isFailedGeomCyclicFit.fit.txt', table = reshape([x, y], [size(x), 2])))    error stop 'table output failed.'")
                        if (0 /= getErrTableWrite(file = 'isFailedGeomCyclicFit.fit.txt', table = reshape([x, y], [size(x), 2])))    error stop 'table output failed.'
        call disp%skip()
    end block
 
end program example

Example Unix compile command via Intel ifort compiler ⛓

#!/usr/bin/env sh
rm main.exe
ifort -fpp -standard-semantics -O3 -Wl,-rpath,../../../lib -I../../../inc main.F90 ../../../lib/libparamonte* -o main.exe
./main.exe

Example Windows Batch compile command via Intel ifort compiler ⛓

del main.exe
set PATH=..\..\..\lib;%PATH%
ifort /fpp /standard-semantics /O3 /I:..\..\..\include main.F90 ..\..\..\lib\libparamonte*.lib /exe:main.exe
main.exe

Example Unix / MinGW compile command via GNU gfortran compiler ⛓

#!/usr/bin/env sh
rm main.exe
gfortran -cpp -ffree-line-length-none -O3 -Wl,-rpath,../../../lib -I../../../inc main.F90 ../../../lib/libparamonte* -o main.exe
./main.exe

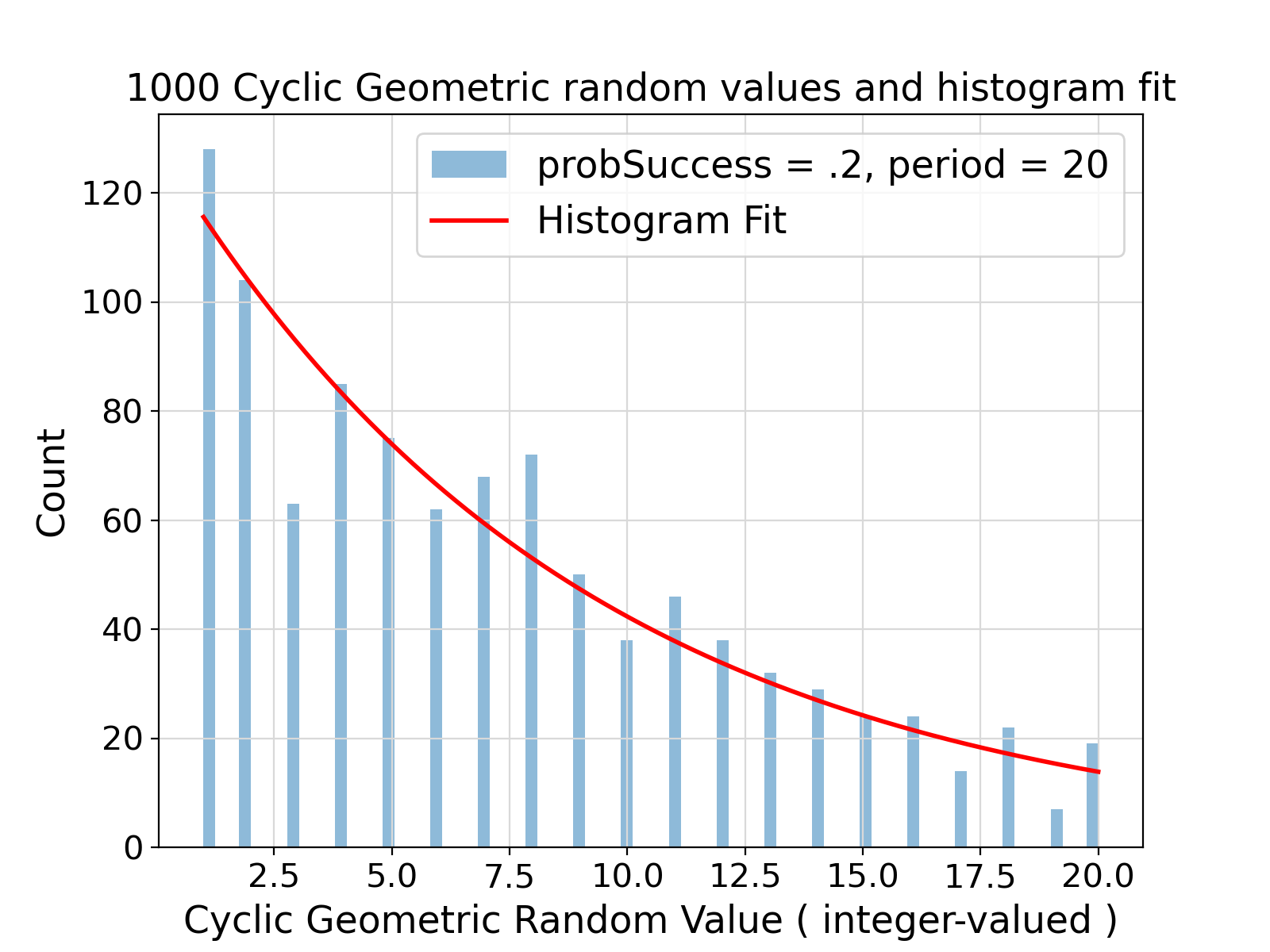
Example output ⛓

 
probSuccess = .1; period = 20
rand = getGeomCyclicRand(probSuccess, [(period, i = 1, 1000)])
if (0 /= getErrTableWrite(file = 'isFailedGeomCyclicFit.rnd.txt', table = rand)) error stop 'table output failed.'
call setUnique(getGeomCyclicRand(probSuccess, [(period, i = 1, 1000)]), unique = stepSuccess, count = freqSuccess, order = -1_IK)
stepSuccess
+1, +2, +4, +3, +5, +6, +8, +9, +7, +11, +12, +10, +13, +14, +15, +17, +16, +20, +18, +19
freqSuccess
+114, +97, +91, +90, +84, +76, +55, +55, +52, +51, +36, +33, +31, +26, +25, +24, +23, +15, +12, +10
if (isFailedGeomCyclicFit(stepSuccess, freqSuccess, period, probSuccessFit, normFacFit)) error stop 'Fitting failed.'
[probSuccessFit, normFacFit]
+0.105651955925097061021975721637957888, +976.572977671411732916580527403386634
x = getLinSpace(1._RKG, real(period, RKG), 500_IK) ! for visualization.
y = normFacFit * probSuccessFit * (1 - probSuccessFit)**(x - 1) / (1 - (1 - probSuccessFit)**period) ! for visualization.
y = normFacFit * exp(getGeomCyclicLogPMF(x, probSuccessFit, period)) ! for visualization.
if (0 /= getErrTableWrite(file = 'isFailedGeomCyclicFit.fit.txt', table = reshape([x, y], [size(x), 2])))    error stop 'table output failed.'
 
 

Postprocessing of the example output ⛓

#!/usr/bin/env python
 
import matplotlib.pyplot as plt
import pandas as pd
import numpy as np
import glob
import sys
 
linewidth = 2
fontsize = 17
 
marker ={ "CK" : "-"
        , "IK" : "."
        , "RK" : "-"
        }
label = [ r"probSuccess = .2, period = 20"
        , r"Histogram Fit"
        ]
 
fig = plt.figure(figsize = 1.25 * np.array([6.4, 4.8]), dpi = 200)
ax = plt.subplot()
 
dfrnd = pd.read_csv("isFailedGeomCyclicFit.rnd.txt", delimiter = ",", header = None)
for j in range(len(dfrnd.values[0,:])):
    plt.hist( dfrnd.values[:,j]
            , histtype = "stepfilled"
            , density = False
            , alpha = 0.5
            , bins = 75
            )
dffit = pd.read_csv("isFailedGeomCyclicFit.fit.txt", delimiter = ",", header = None)
for j in range(1, len(dffit.values[0,:])):
    plt.plot( dffit.values[:, 0]
            , dffit.values[:,j]
            , linewidth = 2
            , c = "red"
            )
    ax.legend   ( label
                , fontsize = fontsize
                )
plt.xticks(fontsize = fontsize - 2)
plt.yticks(fontsize = fontsize - 2)
#ax.set_xlim(0, 10)
ax.set_xlabel("Cyclic Geometric Random Value ( integer-valued )", fontsize = 17)
ax.set_ylabel("Count", fontsize = 17)
ax.set_title("{} Cyclic Geometric random values and histogram fit".format(len(dfrnd.values[:, 0])), fontsize = 17)
 
plt.grid(visible = True, which = "both", axis = "both", color = "0.85", linestyle = "-")
ax.tick_params(axis = "y", which = "minor")
ax.tick_params(axis = "x", which = "minor")
 
plt.savefig("isFailedGeomCyclicFit.png")

Visualization of the example output ⛓

Test:

test_pm_distGeomCyclic

Final Remarks ⛓

---

If you believe this algorithm or its documentation can be improved, we appreciate your contribution and help to edit this page's documentation and source file on GitHub.
For details on the naming abbreviations, see this page.
For details on the naming conventions, see this page.
This software is distributed under the MIT license with additional terms outlined below.

1. If you use any parts or concepts from this library to any extent, please acknowledge the usage by citing the relevant publications of the ParaMonte library.
   
2. If you regenerate any parts/ideas from this library in a programming environment other than those currently supported by this ParaMonte library (i.e., other than C, C++, Fortran, MATLAB, Python, R), please also ask the end users to cite this original ParaMonte library.
   

This software is available to the public under a highly permissive license.
Help us justify its continued development and maintenance by acknowledging its benefit to society, distributing it, and contributing to it.

Copyright

Computational Data Science Lab

Author:

Amir Shahmoradi, Monday March 6, 2017, 3:22 pm, Institute for Computational Engineering and Sciences (ICES), The University of Texas at Austin.

Definition at line 2555 of file pm_distGeomCyclic.F90.

---

The documentation for this interface was generated from the following file:

• src/fortran/main/pm_distGeomCyclic.F90